Static Force Systems

In our previous post, we looked at some basic terminology. In this post, we will look at the mechanics fundamentals and then solve a basic force problem.

Forces may be described as "contact" or "body" forces.

Contact forces are those produced by physical contact between two bodies. The force that a beam exerts on its support girders would be classified as a contact force.

Body forces are those that are the result of the location of the body within some type of field. The force an electric motor rotor winding feels as it passes through the stator field is an example of a body force.

Forces may also be described by how they are applied.
Forces applied over a tiny area relative to the system are called concentrated forces.

Forces that are applied over an area that is large in comparison to the system in question are called distributed forces.

Now that we've established more vocabulary let's look at a vital theorem to consider that will help us in later problems: the Principle of Transmissibility.

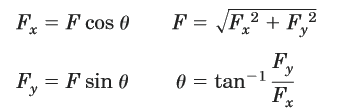
The Principle of Transmissibility states that a force may be applied at any point along its line of action without altering the effects of any external force acting on a rigid body. This allows us to treat any force that meets those criteria as a sliding vector and can safely ignore the application point. This dramatically simplifies calculations.
Speaking of calculations, let's jump into a little bit of math.
Here's a problem to consider:

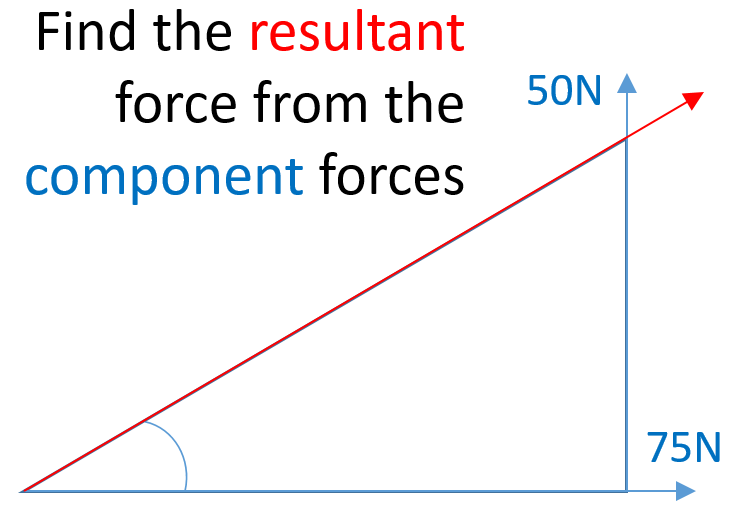
In this case, we'll use the equations on the right-hand side of the list posted just above.
Fnet = sqrt(50²+75²) = 90.138N
theta = arctan (50/75) = 33.690 degrees

As a result, adding a 50N vertical force and a 75N horizontal force will give us a 90.1N force at an angle of 33.7 degrees. We used the Pythagorean Theorem and some quick trigonometry to figure that out.
Let's check our work using the left-hand equations. Don't forget to set your calculator to degree mode first!
Fx = 90.1 cos(33.7) = ~50N
Fy = 90.1 sin(33.7) = ~75N
That was pretty easy, wasn't it?

Another way to write those vector components is <50,75> in vector notation or 50i+75j if you use i-j-k notation.

Check out our next post in this series on Moments and Torque.